XY spin quartets
| >>Code(ZipFile) >>Download DemoVideo |
When studied in two dimensions this results in an interesting
topological structure that consists of vortices and
anti-vortices, located on a basic plaquette of 4 neighboring
lattice sites. Visually, these structures are hard to find in the raw
data, and numerical treatment, e.g. cooling, that brings the
structures to the foreground, also destroys information. The
sonification uses the spin quartet values (looped and
distorted) for modulating the phase of a basic
frequency, which is filtered out in the end. Only interesting structures
remain heard and everything else falls automatically silent. (xy-listening: Demo videos plus description for the XY model sonification.) |
The planar XY-model is a spin model in 2 dimensions, where the degrees of freedom are spins between 0 and 2π .
The XY-model exhibits an interesting topology: the spins form vortices and anti-vortices (a vortex consists of 4 spins, a spin quartet, that rotates by 2 π if followed in the counterclockwise direction. An anti-vortex rotates by - 2 π .) Depending on the temperature as model parameter, the behaviour of vortices and anti-vortices, the topological structures, changes. We find a phase transition, named after Kosterlitz and Thouless. Below the transition, vortex-anti-vortex-pairs stay close together. At higher temperatures, we find a free plasma of vortices and anti-vortices.
In a visualization of this model, our eyes are distracted by fluctuating spins. Only the so-called cooling makes structures visible, but it smoothes the whole configuration and destroys physical information.

Research questions.
How to distinguish audially between rotations in one or the other direction?
How to display spatial topology acoustically?
Is the difference between the high- and the low- temperature phase hearable?
And in general: how to sonify a 2-dimensional system, without sticking to visual concepts?
Our sonification.
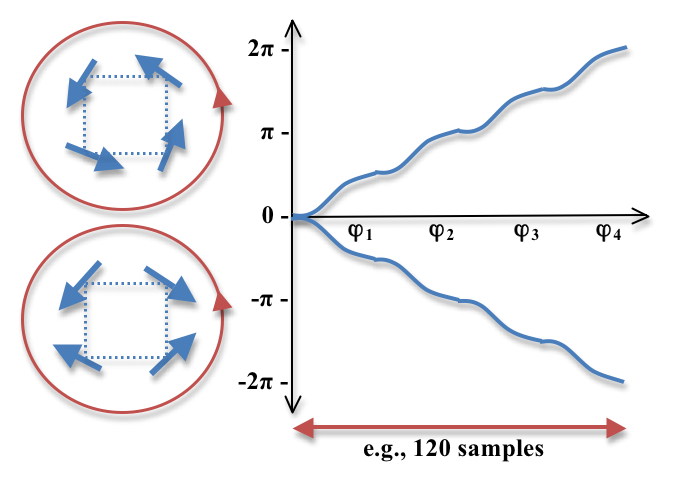
Our approach is based on simple acoustical principles.
We read out the values of spin quartets, and use them as the phase of a sine oscillator at a certain base frequency. In the case of a (anti-)vortex, the phase rises (decreases) by 2 π . The phase is looped and a new frequency results: increased for the vortex, decreased for the anti-vortex. If the spin quartet is rather laminar, the accumulated rotation will add up to 0, and only the base frequency is heard. This frequency is filtered in the end - thus only the interesting topological structures are hearable.
In order to facilitate spatial orientation in the lattice, this information is encoded in multiple ways. A graphical user interface is used for interaction. The distance to the clicking point is mapped over the amplitude and length of the tone (and the resulting macro-rhythm), left-right panning and the changing of the base frequency.
As the base frequencies are mistuned to each other with increasing distance, the vortex- anti-vortex - pairs that are close to each other sound harmonical, while those that are farther produce a beating.
More information can be found at publications
.
>> More Videos With Explanation

 Previous:
Ising gestalts
Previous:
Ising gestalts