Home
QCD waveguides
  |
>>Code |
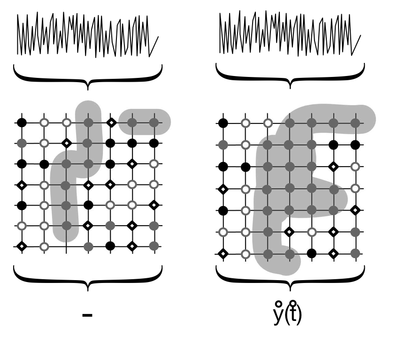
A completely different data set from lattice QCD deals with center symmetry considerations derived from the Polyakov loop. The data are three-dimensional and occur in four different states. The configuration forms clusters, sites of identical states that are connected through links with same state Polyakov loops at their endpoints. A specific phenomenon is percolation, meaning that a cluster ranges from one lattice side to the opposite end. The size of the clusters and whether they percolate depends on the temperature. In this sonification approach, we used a model in which the clusters of each state are regarded as caves of free wave propagation; the borders of the cave defined as hard reflections between different states. The ‘caves’ are excited by white noise or by an impulse at one side of the lattice, and the resulting resonant signal is recorded at the opposite side. The sound signal propagates through the lattice only if there is percolation. The model is based on a 3d waveguide mesh. |
Click on image for audio example:
percolating tunnel with 1x1 sites: 
percolating tunnel with 9x9 sites: 
perclating tunnel with 15x15 sites:
Previous:
Lattice QCD - Topological objects
